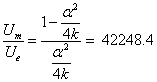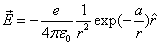
As we know, for the electron point model, there are singularity problems. In order to avoid the singularity problem, let us assume that the electron electromagnetic model is as follows:
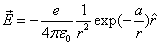
Where e is electron’s electric charge unit,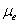 
is the electron magnetic moment.
g is the magnetic charge unit defined as:
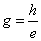
Electron Electric charge
Since we know the electric charge density:
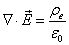
Then we have
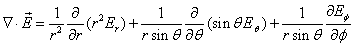
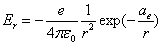
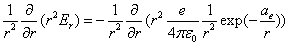
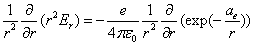
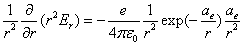
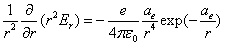
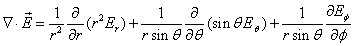
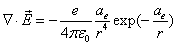
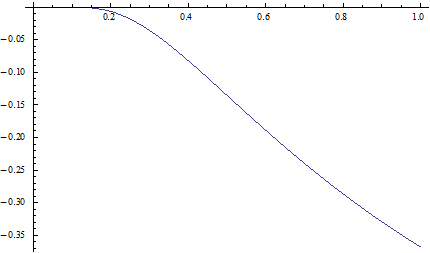
Thus we get the electron’s electric charge density distribution as:
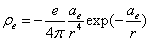
X coordinate is the radius with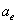 
as the distance unit
Y coordinate is the electric charge density with e as the electric charge unit.
The electric charge within the sphere of radius r is as follows:
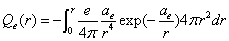
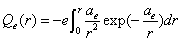
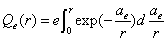
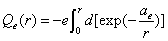
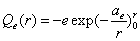
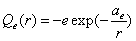
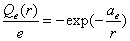
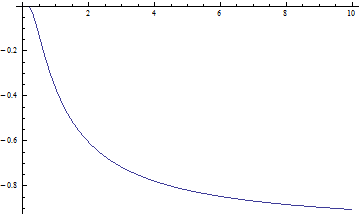
X coordinate is the radius with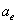 
as the distance unit
Y coordinate is the electric charge density with e as the electric charge unit.
When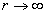 
The electron as a whole has one unit negative electric charge.
Electron Magnetic Charge
Given the magnetic charge density is:
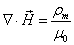
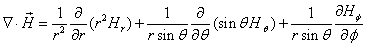
Then the electron magnetic charge is as the following
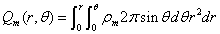
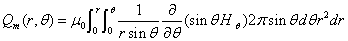
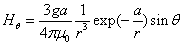
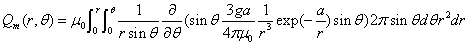
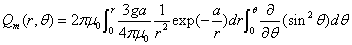
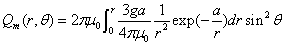
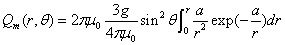
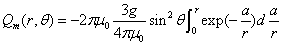
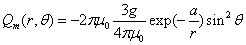
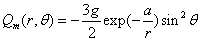
The above equation is the magnetic charge distribution equation inside the electron.
When
and 
We get electron magnetic charge of northern hemisphere as:
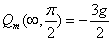
The electron northern hemisphere has a magnetic charge of 3/2g
When
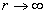
and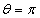 
We get
The electron as whole has zero magnetic charge.
The electron’s electromagnetic field angular momentum
The electromagnetic field angular momentum density is defined as:
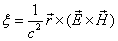
Below is the electromagnetic angular momentum:
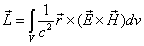
Using the electron electromagnetic field equation, we can get the following:
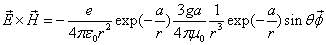
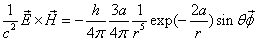
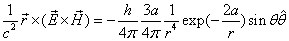
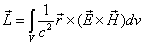
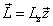
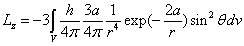
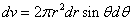
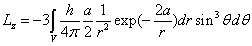
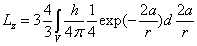
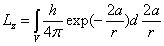
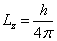
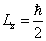
Based on our electron model, the electron always has half spin, the electron spin has an electromagnetic origin and the electron spin is the electromagnetic field angular moment.
Electron’s magnetic moment
The potential energy of the electron magnetic moment in a z direction magnetic field is as follows:
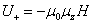
The potential energy of the electron magnetic moment in a negative z direction magnetic field is as follows:
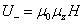
Thus we have
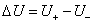
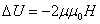
As we also know:
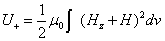
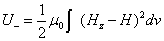
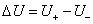
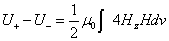
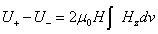
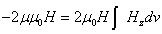
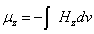
Thus we can get the electron magnetic moment as
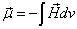
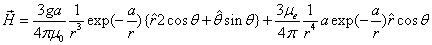
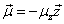
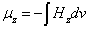
As we know
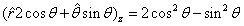
When
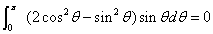
Then
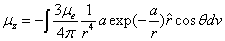
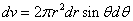
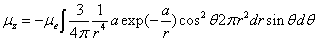
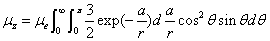
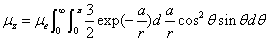
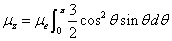
Thus
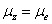
Electron’s Electric field energy
The electric field energy density is as follows:
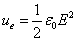
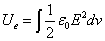
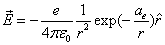
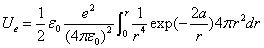
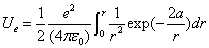
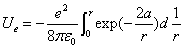
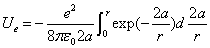
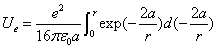
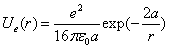
X coordinate is the radius with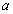 
as the distance unit
Y coordinate is the electric energy with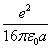 
as the energy unit.
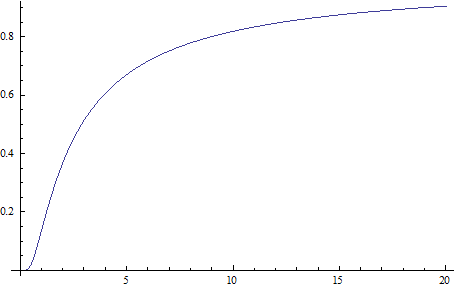
When
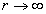
We can get electron’s total electric field energy as
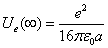
Think of the electron as a capacitor C, thus we have
From the above, we get the electron capacitor as
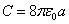
Electron’s magnetic field energy
The magnetic field energy density is:
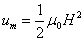
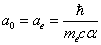
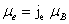
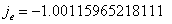
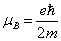
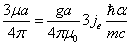
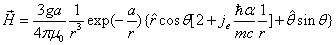
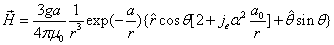
The electron’s magnetic field energy is as the following
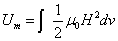
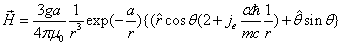
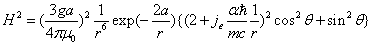
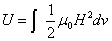
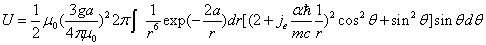
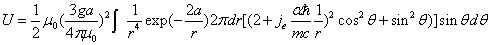
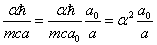
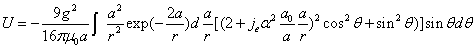
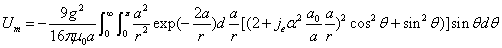
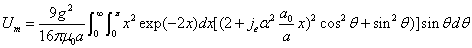
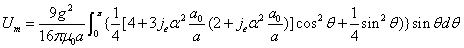
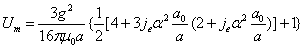
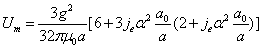
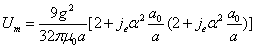
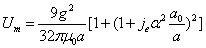
This is the electron’s magnetic field energy
The electron’s electric field energy then becomes
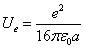
So we get the electron’s electromagnetic field energy as
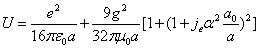
Let us define
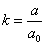
Then we have
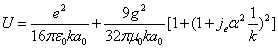
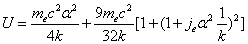
Let us assume that the electron’s mass has an electromagnetic origin, thus we have
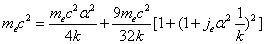
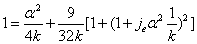
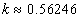
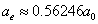
For the electron, the ratio of the magnetic energy to the electric field energy is
